Freecell Game Numbers

The Freecell game as we know it today was invented in 1978 by Paul Alfille for the PLATO computer system and rediscovered and popularized a few years later by Jim Horne, who introduced it for Microsoft's DOS operating system. He was also the one who came up with an algorithm to generate the well-known game numbers, giving the first game he developed 32,000 different numbers. The convenience of these numbers was mainly that the player could remember a number and replay their favorite game.

In offices where solitaire games like this were and still are very popular, entire Freecell tournaments were held on the boss's time. For example, someone would call out a game number and whoever solved the puzzle first was the winner.

Freecell's unbeatable game: number 11982

Dave Ring's Internet Freecell Project greatly increased the popularity of the game. The goal of this project was to solve all 32,000 deals. In August 1994, 100 volunteers from puzzle and math-related websites were enlisted. Each was assigned 100 consecutive deals and worked their way through them. Unsolved cases were passed on to the best solvers. By April 1995, all but one deal had been solved — the infamous number 11982. Despite numerous attempts by players and at least eight independent computer programs, this deal remains unsolved to this day.

Easter egg

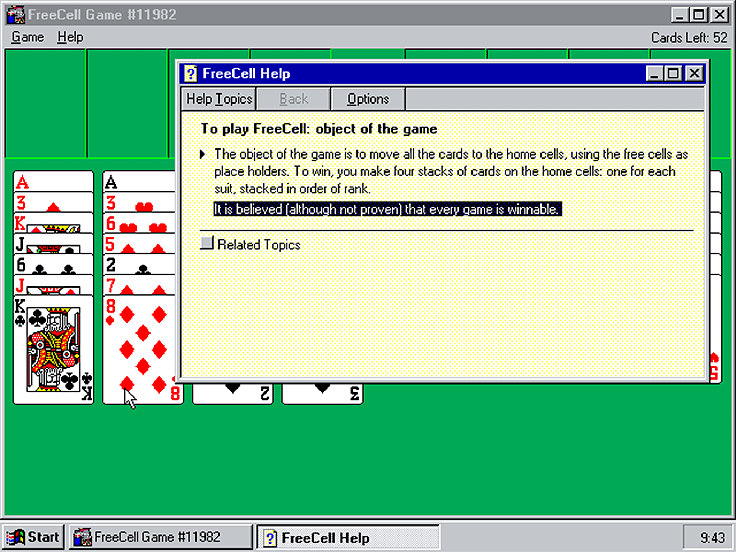Windows 95 was launched after Windows 3.1, and it also included the Freecell game with its well-known 32,000 different deals. In the game's help file, the statement read: "It is believed, although not proven, that every game is winnable." At the time Jim Horne wrote this claim, he was unaware of the unsolvable deal number 11982.

As a joke, the Windows 95 version included two unsolvable deals with the numbers -1 and -2. Horne had deliberately made his claim ambiguous to challenge people to find such impossible deals, but he actually meant that all 32,000 included deals were winnable. This was almost true.

1 million

Over time, the games became more beautiful in design and also received more and more game numbers. Freecell on Windows XP had 1 million different game numbers, all still generated with Jim Horne's algorithm. Of the 1 million, in addition to number 11982, there are another seven that are unwinnable. Of the first million, 0.0008% cannot be solved, so the chance of encountering a deal during that you cannot complete is very small.

From difficult to easy

Through these game numbers, entire communities and forums emerged on the internet, with questions about how to solve a particular number. As a result, it became increasingly clear which games were very easy to solve and which were very difficult. The easiest are the so-called Zero-Freecell games. the zero stands for null, meaning they can be played without using a single free cell. Here's a small selection of the many numbers where this is possible: 892, 5055, 17772, 22663, 25904 and 27121.

Of course, there are also games that are very difficult that require puzzling for hours to solve. Some of these games are: 1443, 14027, 18947, 20725, 21454, 25599, 31302, and 31729. These very difficult games, sometimes requiring a few hundred steps to solve, while the simple ones only need dozens of steps. Out of the first 32,000 deals, there are three games that can be solved in 19 moves if done correctly: 31364, 10913, and 11853. The deal that requires the most moves is 29596 — if done correctly in one attempt, it can be solved in 53 moves.

On our website, we also offer online games where these numbers can be used. Why not try some of these numbers on Microsoft Freecell?